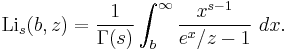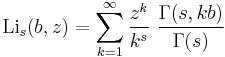Incomplete polylogarithm

In mathematics, the Incomplete Polylogarithm function is related to the polylogarithm function. It is sometimes known as the incomplete Fermi–Dirac integral or the incomplete Bose–Einstein integral. It may be defined by:

$\operatorname{Li}_s(b,z) = \frac{1}{\Gamma(s)}\int_b^\infty \frac{x^{s-1}}{e^x/z-1}~dx.$

Expanding about z=0 and integrating gives a series representation:

$\operatorname{Li}_s(b,z) = \sum_{k=1}^\infty \frac{z^k}{k^s}~\frac{\Gamma(s,kb)}{\Gamma(s)}$

where Γ(s) is the gamma function and Γ(s,x) is the incomplete gamma function.

References